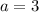Answer:
the coefficient of squared term in the parabola's equation is
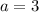
Explanation:
We are given the following information in the question:
Vertex of parabola: (2,-4)
Also, (x = 3, y = -1) lies on the parabola.
The general equation of parabola is of the form:
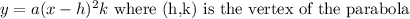
Putting h = 2, k = -4 in the equation, we get:
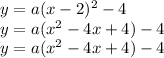
Now, putting x = 3 and y = -1 in the above equation,we get:
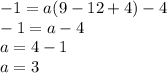
Hence, the coefficient of squared term in the parabola's equation is