The surface area (SA) of a cube can be written as:
SA = 6s²
From here we can write, the length of the side s as:
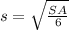
For cube with surface area of 1200 square inches, the side length will be:
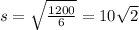
inches
For cube with surface area 768 square inches, the side length will be:
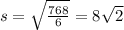
inches
The difference in side lengths of two cubes will be:
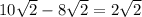 Rounding to nearest tenth of an integer, the difference between the side lengths of two cubes will be 2.8 inches.
Rounding to nearest tenth of an integer, the difference between the side lengths of two cubes will be 2.8 inches.