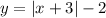we have that
the original function
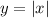 has the vertex at point
has the vertex at point
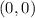
The transformed function has the vertex at point

so
the rule of the translation is equal to

That means
The translation is
 units to the left and
units to the left and
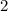 units down
units down
therefore
the answer is
the transformed function is