The distance of the loops form the following sequence:
2.40, 2.44, 2.48, 2.52, 2.56, ...
If we observe these terms, we'll see that the difference between two consecutive terms is the same i.e 0.04. Such a sequence is known as Arithmetic sequence.
We have to find the radius of 95th loop. This means we have to find the 95th term of the sequence.
Using the formula of Arithmetic Sequence, we can write:
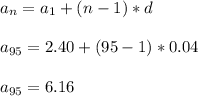
Thus, the radius of 95th loop of paper will be 6.16 centimeters.
So, the correct answer is option B