Answer:

Explanation:
We are given the following information in the question:
A rectangular box has a length of x feet, a width of y feet, and a height of z feet.
Area of base =
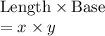
Cost of building base = $4.20 per square foot
Total cost of building base =
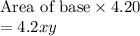
Area of 4 walls =
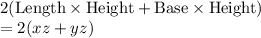
Cost of building base = $0.75 per square foot
Total cost of building 4 walls =
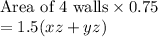
Total cost of building box =
