Answer :
(a) The energy is,

(b) The wavelength (in nm) is,
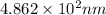
Explanation :
The general formula for the wavelength of spectral line emitted by a hydrogen atom, when it makes a transition from
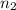 shell to
shell to
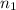 shell is,
shell is,
![(1)/(\lambda)=R_H[(1)/((n_1)^2)-(1)/((n_2)^2)]](https://img.qammunity.org/2020/formulas/chemistry/college/x11r4536gh0pqw0446ijm5f8bano2z7tc7.png)
where,
 = wavelength
= wavelength
Rydberg's Constant =
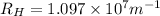
In this,
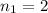 and
and
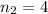
![(1)/(\lambda)=(1.097* 10^7)* [(1)/((2)^2)-(1)/((4)^2)]](https://img.qammunity.org/2020/formulas/chemistry/college/x4p523hj5vmkw766sjp88vq7pdm5achvsp.png)

conversion used :
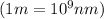
Now we have to calculate the energy.
Formula used :

 = Wavelength =
= Wavelength =
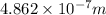
E = energy = ?
c = speed of light =
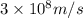
h = Planck's constant =
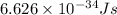
Now put all the given values in the above formula, we get:
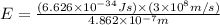
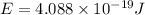
Therefore, the energy is
