Answer:
Path followed by the particle is an incomplete ellipse whose points do not lie on first quadrant.
Explanation:
x = 1+sint
y = 6+2cost
sint = x-1
cost = (y-6)/2
We know
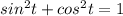
Substituting for sint and cost in the above equation,we get:
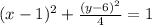
The above equation represents an ellipse with center at (1,6) with major axis length as 4 and minor axis length as 2.
But since π/2 ≤ t ≤ 2π
It represents an incomplete ellipse which does not have any points on the first quadrant.