Question

A 110. g wooden block is initially at rest on a rough horizontal surface when a 12.8 g bullet is fired horizontally into (but does not go through) it. After the impact, the block–bullet combination slides 6.5 m before coming to rest. If the coefficient of kinetic friction between block and surface is 0.750, determine the speed of the bullet (in m/s) immediately before impact.

Answer 1

94 ms⁻¹

Step-by-step explanation:

`V` = Speed of the bullet-block combination after collision

`d` = distance traveled by the combination before coming to stop = 6.5 m

`\mu` = Coefficient of kinetic friction = 0.750

acceleration due to friction on a flat surface can be given as

`a = - \mu g = - (0.750) (9.8) = - 7.35 ms^(-2)`

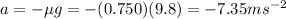
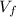`V_(f)` = Final speed of the combination = 0 m/s

Based on the above equation , we can use the kinematics equation as

`V_(f)^(2) = V^(2) + 2 a d\\(0)^(2) = V^(2) + 2 (- 7.35) (6.5)\\V = 9.8 ms^(-1)`

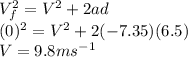
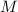`M` = mass of the wooden block = 110 g

`m` = mass of the bullet = 12.8 g

`v` = Speed of the bullet before collision

Using conservation of momentum for inelastic collision , we have

`m v = (m + M) V\\(12.8) v = (12.8 + 110) (9.8)\\v = 94 ms^(-1)`