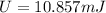Answer:
The answer is 10.857mJ
Step-by-step explanation:
The energy stored in this solenoid is given by the below mentioned equation,
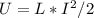
where L the inductance of this solenoid is given by the below mentioned equation,
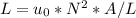
Plugging this into the energy equation you obtain the equation for the total energy stored in the magnetic field of the solenoid, given by,
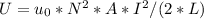
where
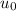 is the permeability of free space which equals to
is the permeability of free space which equals to
 . Plugging all the quantities into the above equation from the data in the question after converting to standard units. of meters instead of centimeters, we get for the energy stored in the coil,
. Plugging all the quantities into the above equation from the data in the question after converting to standard units. of meters instead of centimeters, we get for the energy stored in the coil,