Answer:
0.561 is the probability that the sample mean of their weights lies between 163 and 170.
Explanation:
We are given the following information in the question:
Mean, μ = 167
Standard Deviation, σ = 27
Since the sample size is large, by central limit theorem the distribution of means is a normal distribution.
We are given that the distribution of weights of a population of workers is a bell shaped distribution that is a normal distribution.
Formula:
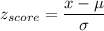
Standard error due to sampling:
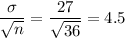
P(weights lies between 163 and 170)
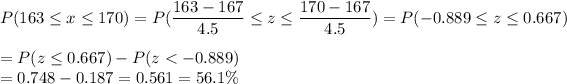
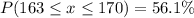
0.561 is the probability that the sample mean of their weights lies between 163 and 170.