Option B
Trevor isn't correct because -2i must also be a root
Solution:
For the polynomial with roots -7, 2i and 7 their roots can be,
1. ) Real roots
2.) Imaginary roots
The real roots are: -7 and +7
The imaginary root given is: 2i
The imaginary roots come from the square root. So they will be in form of
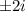
Therefore,
For f(x) with roots -7 and +7 and
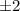 we have,
we have,

Fundamental Theorem of Algebra states that a polynomial will have exactly as many roots as its degree (the degree is the highest exponent of the polynomial).
So for f(x) with 4 roots, degree of f(x) is 4
So option B is correct. Trevor is not correct because –2i must also be a root.