Answer:
The direct variation equation can be given as:
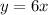
Explanation:
Given:
At a summer camp there are 6 campers under one counselor.
To find the direct variation equation for the number of campers in terms of number of counselor.
Solution:
 Number of campers
Number of campers
 Number of counselors
Number of counselors
We have
 ∝
∝

The direct variation equation can be written as:

where
 is the direct variation constant.
is the direct variation constant.
There are 6 campers under one counselor. Using this statement we can find value of

Given: when
 then
then
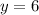
We have,
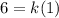
∴
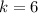
Thus, the direct variation equation can be given as:
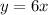
We can find the points using the equation to plot.

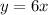
0 0
1 6
2 12
The graph is sown below.