Option A
If f(x) =
 and g(x) =
and g(x) =
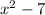 then
then

Solution:
Given that f(x) =
 and g(x) =
and g(x) =
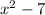
To find: (f - g)(x)
We know that,
(f – g)(x) = f (x) - g(x)
Let us substitute the given values of f(x) and g(x) in above formula,
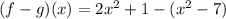
For solving the brackets in above expression,
There are two simple rules to remember:
When you multiply a negative number by a positive number then the product is always negative.
When you multiply two negative numbers or two positive numbers then the product is always positive.
So the expression becomes,
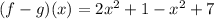
Combining the like terms,

Thus option A is correct