An equation is given. (Enter your answers as a comma-separated list. Round terms to three decimal places where appropriate. If there is no solution, enter NO SOLUTION.) cos θ 2 …

Question

asked May 1, 2020 115k views

1 Answer

Maks Matsveyeu · Answer 1 · 2020-05-06T18:08:30+0000

Answer:

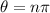
$\theta = n\pi$

where
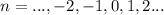
n = ..., -2,-1,0,1,2...

Explanation:

given equation is:

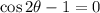
$cos(2\theta) - 1 = 0$

since no range is provided we can solve for all values of
$\theta$ :

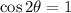
$cos(2\theta) = 1$

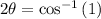
$2\theta = \cos^(-1){(1)}$

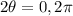$2\theta = 0, 2\pi$ for one cycle of cos
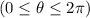
$(0 \leq \theta \leq 2\pi)$

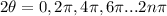$2\theta = 0, 2\pi, 4\pi, 6\pi ... 2n\pi$ for all cycles of cos

we should also include negative values.

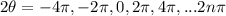
$2\theta = -4\pi,-2\pi,0, 2\pi, 4\pi,... 2n\pi$

we can divide each value by 2, to get the solutions for
$\theta$ instead of
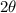
$2\theta$

Answer:

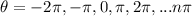
$\theta = -2\pi,-\pi,0, \pi, 2\pi,... n\pi$

This is the solution of the equation
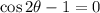$cos(2\theta) - 1 = 0$ .

In its most general form we can write all solutions of the equation in terms of

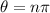$\theta = n\pi$ where
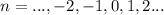n = ..., -2,-1,0,1,2... or n is an integer.