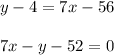Question

(8,4); m=7 what’s the answer in point-slope form

Answer 1

The equation of line in point slope form is y - 4 = 7x - 56

Solution:

Given that m = 7 and point is (8, 4)

We have to find the equation of line in point slope form

It emphasizes the slope of the line and a point on the line

The point slope form is given as:

`y - y_1 = m(x - x_1)`

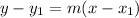
Where "m" is the slope of line

Substitute m = 7 and (x, y) = (8, 4) in above point slope form

`y - 4 = 7(x - 8)`

`y - 4 = 7x - 56`

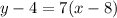
Thus equation of line in point slope form is found

We can write the equation in standard form

The standard form of an equation is Ax + By = C. In this kind of equation, x and y are variables and A, B, and C are integers.

`y - 4 = 7x - 56\\\\7x - y -52 = 0`