Answer:
The required image line is 3x + y = 8
Explanation:
We have to find two points on the given straight line then find their reflection points over the x-axis and then finally the straight line passing through those two image points will give the required straight line.
Now, the given straight line is y = 3x - 8.
Now, two any points on this straight line are say (1,-5) and (2,-2).
So, the image of (1,-5) point reflecting over the x-axis will be (1,5) and the image of the point (2,-2) reflecting over the x-axis will be (2,2).
Therefore, the straight line passing through those two image points will have equation
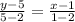
⇒ y - 5 = 3(1 - x)
⇒ y - 5 = 3 - 3x
⇒ 3x + y = 8
Hence, the required image line is 3x + y = 8 (Answer)