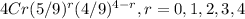Question

A bag contains 1 red, 3 green, and 5 yellow balls. A sample of four balls is picked. Let G be the number of green balls in the sample. Let Y be the number of yellow balls in the sample. Find the conditional probability mass function of G given Y " 2 assuming the sample is picked with replacement.

Answer 1

P(Y=r) =
`4Cr (5/9)^r (4/9)^(4-r),r=0,1,2,3,4`

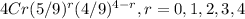
Explanation:

Given that a bag contains 1 red, 3 green, and 5 yellow balls. A sample of four balls is picked, with replacement

When balls are picked with replacement, each time the ball picked up does not depend on the previous outcomes.

Thus given y=2 will not affect the pdf of G

In G, Y no of yellow balls can take values as 0,1,2,3,4

Prob of drawing a yellow ball each time = 5/9

Y = no of yellow balls is binomial with n =4 and p = 5/9

P(Y=0) =
`(1-5/9)^4 = ((4)/(9) )^4`

In general,

P(Y=r) =
`4Cr (5/9)^r (4/9)^(4-r),r=0,1,2,3,4`