Answer:
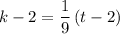 .
.
Explanation:
Note that Ulissa is running at a constant pace. This problem is very similar to finding the equation for a line on an
 -
-
 plane given two points on that line. In this case, time
plane given two points on that line. In this case, time
 (in minutes) acts as the dependent variable and acts as,
(in minutes) acts as the dependent variable and acts as,
 . Distance
. Distance
 acts as the dependent variable,
acts as the dependent variable,
 .
.
Start by finding the slope of that line: given two points on that line
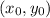 and
and
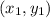 , the slope of that line would be equal to
, the slope of that line would be equal to
 .
.
In this case, the variables are different but the formula shall still apply. Note that the dependent variable
 is on the numerator while the independent variable
is on the numerator while the independent variable
 is on the denominator. Apply the formula to find slope:
is on the denominator. Apply the formula to find slope:
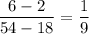 .
.
Apply the slope-point form to any point on the line to obtain the line's equation. Note that this problem provided two points. They will produce two equivalent equations.
The slope-point form of a line with slope
 and a point
and a point
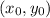 will be:
will be:
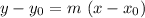 .
.
In this question, applying the formula to the point
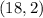 will give the equation:
will give the equation:
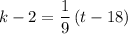 .
.
Similarly, applying the formula to the point
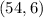 will give the equation:
will give the equation:
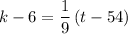 .
.