Question

In an arithmetic sequence:

S14 = -63
a14 = -24
Find the first term, a₁, of the sequence.

Answer 1

a₁ = 15

Explanation:

the sum to n terms of an arithmetic sequence is

`S_(n)` =
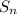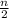`(n)/(2)` [ 2a₁ + (n - 1)d ]

where a₁ is the first term and d the common difference

given S₁₄ = - 63 , then

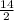`(14)/(2)` [ 2a₁ + 13d ] = - 63

7(2a₁ + 13d) = - 63 ( divide both sides by 7 )

2a₁ + 13d = - 9 → (1)

the nth term of an arithmetic sequence is

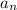`a_(n)` = a₁ + (n - 1)d

given a₁₄ = - 24 , then

a₁ + 13d = - 24 → (2)

subtract (2) from (1) term by term to eliminate d

a₁ + 0 = - 9 - (- 24)

a₁ = - 9 + 24 = 15