The question is missing figure. So, the figure is attached below.
Answer:
29.3
Explanation:
Given:
The given figure is a right angled triangle.
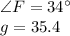
Using the cosine ratio which states that cosine of angle is equal to the ratio of the adjacent side of the angle and the hypotenuse of the triangle.
Here, 'g' is the hypotenuse and 'h' is the adjacent side. So,
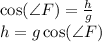
Plug in 35.4 for 'g, 34° for 'F' and solve for 'h'. This gives,

Therefore, the value of 'h' is 29.3.