Answer:
B. [-19.81; -15.35]
Explanation:
Hello!
There was a 95% Confidence Interval for the difference between means of the height of male college basketball players and female basketball players.
15.35 < μ₁ - μ₂ < 19.81
Where
μ₁ is the population mean of the height of male college basketball players
μ₂ is the population mean of the height of female college basketball players
And you are asked if, with the given interval you can calculate another one where the populations will be reversed (i.e. average female heights for μ₁ and average male heights for μ₂)
The formula used to calculate this interval is:
(keep in mind that everything with the subfix 1 will correspond to the males' heights and everything with the 2 subfixes will correspond to the females' heights)
(X[bar]₁ - X[bar]₂) ±
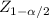* √(σ₁²/n₁ + σ₂²/n₂)
This interval is centered in the difference between the two sample means so you can calculate it from the given interval as:
(X[bar]₁ - X[bar]₂)= (Lower bond + Upper bond)/2
(X[bar]₁ - X[bar]₂)= (15.35 + 19.81)/2
(X[bar]₁ - X[bar]₂)= 17.58
If the distance between "males - females" is 17.58, you could say that the distance between "females - males" would be the same but with opposite direction: -17.58
Now you can clear the margin of error for the interval.
If d is the margin of error, you can calculate it as:
d= (Upper bond - Lower bond)/2 = (19.81 - 15.35)/2 = 2.23
- or -
d=
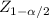* √(σ₁²/n₁ + σ₂²/n₂)
Considering that mathematically the variance of "males - females" ((σ₁²/n₁ + σ₂²/n₂)) will be the same as "females - males" and at the same confidence level of 95%, the Z-value would be the same for the new interval.
Be the structure of the confidence interval: point estimation ± margin of error, the new interval (Females= population 1 and males= population two) will be
(X[bar]₁ - X[bar]₂) ± d
-17.58 ± 2.23
[-19.81; -15.35]
I hope it helps!
The question was incomplete, I've googled it and found the complete question.
If the heights of male college basketball players and female basketball players are used to construct a 95% confidence interval for the difference between the two population means, the result is 15.35 cmless thanmu 1minusmu 2less than19.81 cm, where heights of male players correspond to population 1 and heights of female players correspond to population 2. Express the confidence interval with heights of female basketball players being population 1 and heights of male basketball players being population 2. A. 15.25 cmless thanmu 1minusmu 2less than19.81 cm B. minus19.81 cmless thanmu 1minusmu 2less thanminus15.35 cm C. minus15.35 cmless thanmu 1minusmu 2less thanminus19.81 cm D. This cannot be determined without having the original data values.