Question

A life insurance company has determined that each week (7 days) an average of seven claims is filed in its Nashville branch. Define x as the number of claims filed in a one-week period. What is the probability that during the next 3 days, two claims will be filed?

Answer 1

The probability of having two claims in three days is 0.225.

Explanation:

This type of process can be modeled by the Poisson distribution.

This distribution has a rate, that in this case is x: number of claims filled in a one-week period.

The probabilty density function can be written as

`P(y=k)=\frac{(xt)^ke^(-xt)} {k!}`

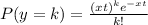
In this case,
`t =3 \,days =3/7\,weeks= 0.429\,weeks`.

The probablity of having two claims in three days is:

`P(y=2)=\frac{(7*(3/7))^2e^(-7*(3/7))} {2!}=(3^2*e^(-3))/(2)=(9*0.05)/(2)=0.225`

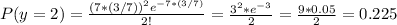
The probability of having two claims in three days is 0.225.