Question

A survey​ asked, "How many tattoos do you currently have on your​ body?" Of the 1221 males​ surveyed, 193 responded that they had at least one tattoo. Of the 1005 females​ surveyed, 130 responded that they had at least one tattoo. Construct a 99​% confidence interval to judge whether the proportion of males that have at least one tattoo differs significantly from the proportion of females that have at least one tattoo. Interpret the interval.

Answer 1

Explanation:

Given that a survey​ asked, "How many tattoos do you currently have on your​ body?" Of the 1221 males​ surveyed, 193 responded that they had at least one tattoo. Of the 1005 females​ surveyed, 130 responded that they had at least one tattoo.

`H_0:p_1 = p_2\\H_a: p_1 \\eq p_2`

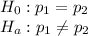
(two tailed test at 1% significance level)

Difference in proportions =
`(193)/(1221) -(130)/(1005) \\=0.029`

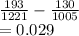
Standard error for difference in proportions = 0.0150

Margin of error = 2.58*std error

= 0.0387

99% confidence interval =

`(0.029-0.0387, 0.029+0.0387)\\=(-0.0097, 0.0677)`

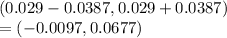
Since this confidence interval contains 0 for difference in proporiton we can say that H0 is true.

We are 99% confidence that for samples randomly drawn for large sizes, the males and females have approximately the same proportion.