Question

In 200​0, a reproductive clinic reported 41 live births to 165 women under the age of​ 38, but only 5 live births for 92 clients aged 38 and older. Is there evidence of a difference in the effectiveness of the​ clinic's methods for older​ women? Complete parts a through c. Use alphaαequals=0.010.01.

Answer 1

Null hypothesis:
`p_(Y) - p_(O)=0`

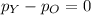
Alternative hypothesis:
`p_(Y) - p_(O) \\eq 0`

`z=\frac{0.248-0.0543}{\sqrt{0.179(1-0.179)((1)/(165)+(1)/(92))}}=3.883`

`p_v =2*P(Z>3.883)=5.15x10^(-5)`

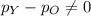
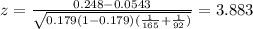
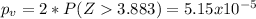
Reject the null hypothesis because there is sufficient evidence to support the claim that there is a difference in the effectiveness of the methods

The 99% confidence interval would be given (0.0877;0.2997).

Explanation:

1) Data given and notation

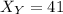`X_(Y)=41` represent the number of live births in women under the age of 38

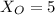`X_(O)=5` represent the number of residents of live births in women aged 38 and older

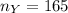`n_(Y)=165` sample of women under the age of 38

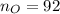`n_(O)=92` sample of women aged 38 and older

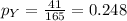`p_(Y)=(41)/(165)=0.248` represent the proportion of live births in women under the age of 38

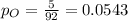`p_(O)=(5)/(92)=0.0543` represent the proportion of live births in women aged 38 and older

z would represent the statistic (variable of interest)

`p_v` represent the value for the test (variable of interest)

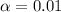`\alpha=0.01` significance level given

2) Concepts and formulas to use

We need to conduct a hypothesis in order to check if there evidence of a difference in the effectiveness of the​ clinic's methods for older​ women, the system of hypothesis would be:

Null hypothesis:
`p_(Y) - p_(O)=0`

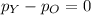
Alternative hypothesis:
`p_(Y) - p_(O) \\eq 0`

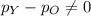
We need to apply a z test to compare proportions, and the statistic is given by:

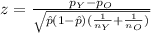`z=\frac{p_(Y)-p_(O)}{\sqrt{\hat p (1-\hat p)((1)/(n_(Y))+(1)/(n_(O)))}}` (1)

Where
`\hat p=(X_(Y)+X_(O))/(n_(Y)+n_(O))=(41+5)/(165+92)=0.179`

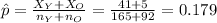
3) Calculate the statistic

Replacing in formula (1) the values obtained we got this:

`z=\frac{0.248-0.0543}{\sqrt{0.179(1-0.179)((1)/(165)+(1)/(92))}}=3.883`

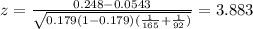
4) Statistical decision

Since is a two sided test the p value would be:

`p_v =2*P(Z>3.883)=5.15x10^(-5)`

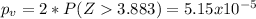
Comparing the p value with the significance level given
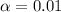`\alpha=0.01` we see that
`p_v<\alpha` so we can conclude that we have enough evidence to reject the null hypothesis, and we can say that there is a significant difference between the two propotions analyzed.

Reject the null hypothesis because there is sufficient evidence to support the claim that there is a difference in the effectiveness of the methods

5) Confidence interval for the difference of proportions

The confidence interval for the difference of two proportions would be given by this formula

`(\hat p_Y -\hat p_O) \pm z_(\alpha/2) \sqrt{(\hat p_Y(1-\hat p_Y))/(n_Y) +(\hat p_O (1-\hat p_O))/(n_O)}`

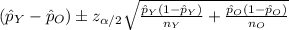
For the 99% confidence interval the value of
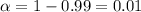`\alpha=1-0.99=0.01` and
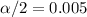`\alpha/2=0.005`, with that value we can find the quantile required for the interval in the normal standard distribution.

`z_(\alpha/2)=2.58`

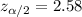
And replacing into the confidence interval formula we got:

`(0.248-0.0543) - 2.58 \sqrt{(0.248(1-0.248))/(165) +(0.0543(1-0.0543))/(92)}=0.0877/tex]   </p><p>[tex](0.248-0.0543) + 2.58 \sqrt{(0.248(1-0.248))/(165) +(0.0543(1-0.0543))/(92)}=0.2997`

And the 99% confidence interval would be given (0.0877;0.2997).

There is 99% confidence that the proportion of successful live births at th clinic is between 8.77% and 29.97% for mothrs under 38 for those 38 and older.