Question

Gabby and Sydney bought some pens and pencils. Gabby bought 4 pens and 5 pencils for $6.71. Sydney bought 5 pens and 3 pencils for $7.12. Find the cost of each.

Answer 1

The cost of each pens is $1.19

The cost of each pencils is $0.39

Explanation:

Given as :

The number of pens bought by Gabby = 4

The number of pencils bought by Gabby = 5

The number of pens bought by Sydney = 5

The number of pencils bought by Sydney = 3

The total cost of Gabby items = $6.71

The total cost of Sydney items =$7.12

Let The cost of each pens = x

And The cost of each pencils = y

Now, According to question

For Gabby

4 x + 5 y = $6.71 .............1

For Sydney

5 x + 3 y = $7.12 .............2

Now, Solving both equations

5 × (4 x + 5 y) - 4 × (5 x + 3 y) = 5 × 6.71 - 4 × 7.12

Or, 20 x + 25 y - 20 x - 12 y = 33.55 - 28.48

Or, (20 x - 20 x) + (25 y - 12 y) = 5.07

or, 0 + 13 y = 5.07

∴ y =
`(5.07)/(13)`

I.e y = $0.39

So, The cost of each pencils = y = $0.39

Again , put The value of y in eq 1

I,e 4 x + 5 y = $6.71

Or, 4 x + 5 × 0.39 = 6.71

or, 4 x + 1.95 = 6.71

or, 4 x = 6.71 - 1.95

or , 4 x = 4.76

∴ x =
`(4.76)/(4)`

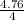
I.e x = $1.19

The cost of each pens = x = $1.19

Hence The cost of each pens is $1.19

And The cost of each pencils is $0.39 Answer