Question

write an equation of a line passing through the point (-1,3) and parallel to AB with A(3,-5) and B (-2,15)

Answer 1

y = -4x - 1

Explanation:

Let's first find the equation of the line segment AB. The equation of a line in slope-intercept form is y = mx + b where m represents the slope and b represents the y-intercept. Start by finding the slope.

Finding the Slope of AB

The equation for slope is:
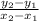`(y_2-y_1)/(x_2-x_1)`. These variables represent any pair of coordinates on the line. In this case since the two points chosen are A and B, thus:

`x_2=-2\\x_1=3\\y_2=15\\y_1=-5`

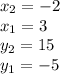
If we plug these values into the equation, we get:

`(15-(-5))/(-2-3)=(20)/(-5)=-4`

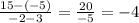
Now we need to find the equation of a line that passes through the point (-1, 3) and is parallel to AB. If two lines are parallel they share the same slope. The equation of the line parallel to AB is y = -4x + b. We can plug in the coordinate (-1, 3) into the equation to solve for b.

Solving for the y-intercept

`y = mx+b\\3 = -4(-1) +b\\3=4+b\\\text{Subtract 4 from both sides}\\b=-1`

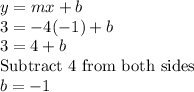
Therefore the equation of the line that passes through the point (-1, 3) and is parallel to AB is y = -4x - 1.