Question

A rock is thrown straight up with an initial velocity of of 24.9m/s^2 . what maximum height will the rock reach before starting to fall downward?

Answer 1

maximum height = 31.63 m

Step-by-step explanation:

Please notice that the units of velocity that you were giving are incorrect. They should be m/s. The ones given in the problem are in fact units of acceleration (not velocity). I am assuming that the initial velocity of the rock is 24.9 m/s to solve this problem.

Let's start by finding the time it takes the rock to reach that maximum height at which point, the velocity of the rock will be zero (just before changing its direction of movement and starting heading down.

We can use the fact that the initial velocity (
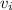`v_i`) of the rock is 24.9 m/s, the final velocity (
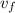`v_f`) at the maximum height is zero, and that the only acceleration it is getting is that of gravity (g) slowing down it motion:

`v_f-v_i=-g\,*\,t\\0-24.9=-9.8\,*\,t\\t=(-24.9)/(-9.8) \\t=2.54\,s`

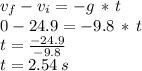
Now we can use this time it takes the rock to reach the maximum height, in the kinematic expression for the distance covered:

`y_f-y_i=v_i\,*\,t-(1)/(2) \,g\,*\,t^2\\y_f-y_i=24.9\,(2.54)-(1)/(2) \,9.8\,*\,(2.54)^2\\y_f-y_i=31.63\,m`

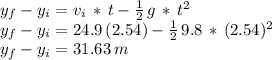
The units of height will come directly in meters (m) after evaluating, since we use all the quantities in the SI system.