Answer:
c) 330 ft by 220 ft with the divider 220 ft long.
Explanation:
We are given that
Let length of playground=y
Breadth of playground=x
Fencing used=1320 ft
We have to find the dimensions of playground that will enclose the greatest total area.
According to question
Fencing used=x+x+x+y+y=3x+2y
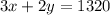
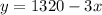
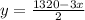
Area of rectangular playground=

Area of rectangular playground=
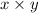
Substitute the values then ,we get
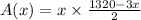
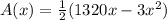
Differentiate w.r.t x
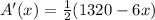
Using formula:
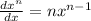
Substitute A'(x)=0
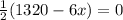
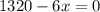
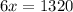

Again differentiate w.r.t x
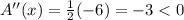
Hence, the area of rectangular playground is maximum at x=220
Substitute x=220
Then,
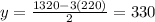
Length of rectangular playground=330 ft
Breadth of rectangular playground=220 ft
Option c is true.
c) 330 ft by 220 ft with the divider 220 ft long.