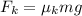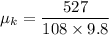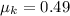Question

A 108 kg clock initially at rest on a horizontal floor requires a 653 N horizontal force to set it in motion. After the clock is in motion, a horizontal force of 527 N keeps it moving with a constant velocity.

(a) Find μs between the clock and the floor.
(b) Find μk between the clock and the floor.

Answer 1

`\mu_s=0.61`

`\mu_k=0.49`

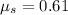
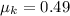
Step-by-step explanation:

Given that,

Mass of the clock, m = 108 kg

When the clock is not moving, force acting on it,
`F_1=653\ N`

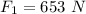
For the clock in motion, force acting on it,
`F_2=527\ N`

To find,

`\mu_s\ and\ \mu_k`

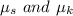
Solution,

When an object is at rest, the force acting on it is called force due to static friction and if the object is in motion, the force acting on its called force due to kinetic friction.

Let
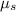`\mu_s` is the coefficient of static friction. Force is given by :

`F_s=\mu_smg`

`\mu_s=(F_s)/(mg)`

`\mu_s=(653)/(108* 9.8)`

`\mu_s=0.61`

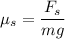
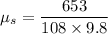
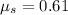
Let
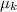`\mu_k` is the coefficient of kinetic friction. Force is given by :

`F_k=\mu_kmg`

`\mu_k=(F_k)/(mg)`

`\mu_k=(527)/(108* 9.8)`

`\mu_k=0.49`