Question

You are buying brats and hot dogs for a barbecue, Brats cost $2.00 per pound and hot

dogs cost $3.00 per pound. You have $36 to spend. Create a linear equation to
represent the different number of combinations of brats and hot dogs you can have,
then write it in slope-intercept form

Answer 1

The linear equation which represent the different number of brats and hot dogs is $ 2 × b + $ 3 × d = $ 36

The slope intercept form of relation is b = 1.5 d + 18

Explanation:

Given as :

The price of Brats = $ 2.00 per pound

The price of hot dogs = $ 3.00 per pound

The total money spend in buying = $ 36

Let The number of Brats = b

And The number of hot dogs = d

Now, According to question

The total money spend on buying = ( The price of Brats × number of Brats ) + ( The price of hot dogs × number of hot dogs )

or, $ 2 × b + $ 3 × d = $ 36

So, The linear equation which represent the different number of brats and hot dogs is $ 2 × b + $ 3 × d = $ 36

Now, in slope intercept form

∵ $ 2 × b + $ 3 × d = $ 36

Or, $ 2 × b = $ 36 - $ 3 × d

Or, $ 2 × b = - $ 3 × d + $ 36

now, comparing with slope intercept , I.e y = m x + c

So, $ 2 × b = - $ 3 × d + $ 36

Or, b =
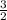`(3)/(2)` × d +
`(36)/(2)`

Or, b = 1.5 d + 18

So, the slope intercept form of relation is b = 1.5 d + 18

Hence , The linear equation which represent the different number of brats and hot dogs is $ 2 × b + $ 3 × d = $ 36

And The slope intercept form of relation is b = 1.5 d + 18 answer