Question

By observing that the centripetal acceleration of the Moon around the Earth is ac = 2.74 × 10-3 m/s2, what is the gravitatonal constant G, in cubic meters per kilogram per square second? Assume the Earth has a mass of ME = 5.97 × 1024 kg, and the mean distance between the centers of the Earth and Moon is rm = 3.81 × 108 m.

Answer 1

`G=6.66* 10^(-11)\ m^3kg^(-1)s^(-2)`

Step-by-step explanation:

The centripetal acceleration of the Moon around the Earth is,
`a_c=2.74* 10^(-3)\ m/s^2`

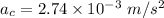
Mass of the Earth,
`M=5.97* 10^(24)\ kg`

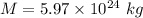
The mean distance between the centers of the Earth and Moon,
`r=3.81* 10^(8)\ m`

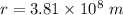
The centripetal acceleration of the Moon around the Earth is given by the formula as :

`a=(GM)/(r^2)`

`G=(ar^2)/(M)`

`G=(2.74* 10^(-3)* (3.81* 10^(8))^2)/(5.97* 10^(24))`

`G=6.66* 10^(-11)\ m^3kg^(-1)s^(-2)`

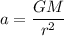
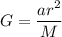
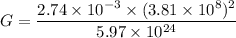
Hence, this is the required solution.