Question

Each of the dimensions of a pyramid are doubled. What is true about the volume of the new pyramid?

The new pyramid has a volume that is
the volume of the original pyramid.
The new pyramid has a volume that is 2 times the volume of the original pyramid.
The new pyramid has a volume that is 4 times the volume of the original pyramid.
The new pyramid has a volume that is 8 times the volume of the original pyramid

Answer 1

The new pyramid has a volume that is 8 times the volume of the original pyramid

Explanation:

we know that

If two figures are similar, then the ratio of its corresponding sides is equal to the scale factor and the ratio of its volumes is equal to the scale factor elevated to the cube

Let

z ----> the scale factor

V_1 -----> volume of the original pyramid

V_2 -----> volume of the new pyramid

`z^(3)=(V_2)/(V_1)`

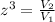
In this problem we have that each of the dimensions of the original pyramid are doubled

so

`z=2`

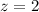
so

substitute

`2^(3)=(V_2)/(V_1)`

`8=(V_2)/(V_1)`

`V_2=8V_1`

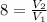
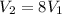
therefore

The volume of the new pyramid is 8 times the volume of the original pyramid