Question

What is the perimeter of the triangle? Round to the nearest tenth.

30.5 Units


12.8 Units


23.5 Units


19.8 Unit

Answer 1

The answer is 19.8

Explanation:

Answer 2

Answer: 19.8 Units

Explanation:

The triangle is shown in the attached figure, and have three vertices:
`AB`,
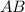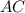`AC` and
`BC`.

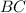Each vertice represents a point in the cartesian plane and the length of each side of the triangle is the modulus of each vector.

In this sense, the modulus
`m` is calculated as shown:

`m=\sqrt{(x_(2)-x_(1))^(2) + (y_(2)-y_(1))^(2)}`

Hence, for each vector:

`AB=\sqrt{(-2-3)^(2) + (3-0)^(2)}=√(34)`

`AC=\sqrt{(-2-(4))^(2) + (3-(-3))^(2)}=√(40)`

`BC=\sqrt{(-4-3)^(2) + (-3-0)^(2)}=√(58)`

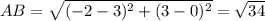
Now, the perimeter of a triangle is the sum of its three sides:

`AB+AC+BC=√(34) + √(40) + √(58)`

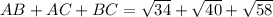
Finally:

`AB+AC+BC=19.77 \approx 19.8`