Answer:
Inductance as calculated is 13.12 mH
Solution:
As per the question:
Length of the coil, l = 12 cm = 0.12 m
Diameter, d = 1.7 cm = 0.017 m
No. of turns, N = 235
Now,
Area of cross-section of the wire, A =
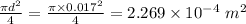
We know that the inductance of the coil is given by the formula:
