Answer:
Explanation:
Given that x is a random variable with probability density function given as
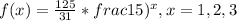
To find cumulative function for x
F(1) =

F(2) =
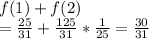
F(3) =
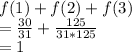
a) P(X lessthanorequalto 1.5)
=
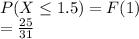
(b) P(X lessthanorequalto 3) =__F(3) = 1_________
(c) P(X > 2) =__f(3) = 1/31________
(d) P(1 < X lessthanorequalto 2) =__f(2) = 25/31____