Question

With food prices becoming a great issue in the world; wheat yields are even more important. Some of the highest yielding dry land wheat yields are in Washington state and Idaho; with an average of 100 bushels per acre and a known standard deviation of 30 bushels per acre. A seed producer would like to save the seeds from the highest 90% of their plantings. Above what yield should they save the seed (bushels/acre)?

80.8

138.4

78.4

73.0

61.6

Answer 1

Option E) 61.6

Explanation:

We are given the following information in the question:

Mean, μ = 100 bushels per acre

Standard Deviation, σ = 30 bushels per acre

We assume that the distribution of yield is a bell shaped distribution that is a normal distribution.

Formula:

`z_(score) = \displaystyle(x-\mu)/(\sigma)`

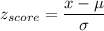
P(X>x) = 0.90

We have to find the value of x such that the probability is 0.90

P(X > x)

`P( X > x) = P( z > \displaystyle(x - 100)/(30))=0.90`

`= 1 -P( z \leq \displaystyle(x - 100)/(30))=0.90`

`=P( z \leq \displaystyle(x - 100)/(30))=0.10`

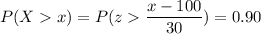
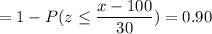
Calculation the value from standard normal table, we have,

`P(z<-1.282) = 0.10`

`\displaystyle(x - 100)/(30) = -1.282\\x = 61.55 \approx 61.6`

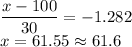
Hence, the yield of 61.6 bushels per acre or more would save the seed.