Question

PLEASE HELP ASAP!!!

What is the approximate area of sector TSV?
Round the answer to the nearest tenth.

A. 3.1 m^2

B. 2.3 m^2

C. 1.1 m^2

D. 0.5 m^2

Answer 1

C) The area of the sector TSV = 1.1 sq m.

Explanation:

Here, the angle subtended by TSV at the center of the circle = 3 radians.

1 Radians = 57.2958°

So, 3 radians = 3 x 57.2958 = 171.887 °

⇒The angle of the sector TSV = 171.887 °

Now, the angle of any sector of a circle with angle Ф at the center

=
`(\theta)/(360)  * \pi * (r^2)`

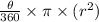
Here, r = 0.87 m

So, the area of the sector TSV =
`(171.887)/(360)  * (22)/(7) * (0.87)^2 = 1.1358`

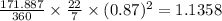
or, the area of the sector TSV = 1.13 sq m.

Now, rounding off the area to the nearest tenth ,we get:

The area of the sector TSV = 1.1 sq m.