Answer:
107,426, bigger
Explanation:
Given that a soccer ball manufacturer wants to estimate the mean circumference of soccer balls within 0.05 in.
Margin of error = 0.05 inches
Since population std deviation is known we can use z critical value.
(a) i.e. for 99% confidence interval
Z critical = 2.58

A minimum sample size of 107 needed.
b)
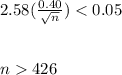
Here minimum sample size = 426
Due to the increased variability in the population, a bigger sample size is needed to ensure the desired accuracy.