Question

HELP PLEASE
What is the starting point and rate of change for
a(x) = 210(1.12)x

Answer 1

Starting point is (0, 210).

Rate of change is 0.12 or 12%.

Explanation:

Given:

The given function is:

`a(x)=210(1.12)^x`

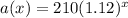
The above function represents an exponential growth function of the form:

`a(x)=a_0(1+r)^x\\Where,\ a(x)\rightarrow \textrm{Value after a given quantity of x}\\a_0\rightarrow \textrm{Initial value or Starting value when 'x' is 0.}\\r\rightarrow \textrm{Rate of change of the value}\\x\rightarrow \textrm{The variable on which the value 'a' depends}`

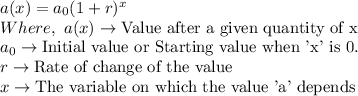
Now, we need to convert the given function in the standard form.

So, we write 1.12 as sum of 1 and a number.

So, 1.12 = 1 + 0.12

Therefore, the given function can be rewritten as:

`a(x)=210(1+0.12)^x`

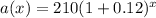
Now, on comparing it with the general form, we conclude:

`a_0=210\\r=0.12`

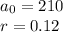
Therefore, the rate of change is 0.12 or 12 %.

Also, the starting point on the graph will be when 'x' is 0. So, the starting point is (0,210).