Answer:
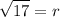
Explanation:
According to the Centre-Radius Formula,
![\displaystyle [X - H]^2 + [Y - K]^2 = R^2,](https://img.qammunity.org/2020/formulas/mathematics/high-school/qt0rpgsl5eajqxepejags8iny95ogerscq.png) [H, K] represents the centre of the circle, where the negative symbols give the OPPOSITE terms of what they really are, and the radius is ALWAYS squared. So, to find the radius of this circle, you just simply plug the coordinates into the equation:
[H, K] represents the centre of the circle, where the negative symbols give the OPPOSITE terms of what they really are, and the radius is ALWAYS squared. So, to find the radius of this circle, you just simply plug the coordinates into the equation:

I am joyous to assist you anytime.