Answer:
65.56°
Explanation:
We know that if we take dot product of two vectors then it is equal to the product of magnitudes of the vectors and cosine of the angle between them
That is let p and q be any two vectors and A be the angle between them
So, p·q=|p|*|q|*cosA
⇒
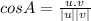
Given u=-8i-3j and v=-8i+8j
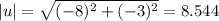

let A be angle before u and v
therefore,
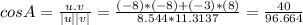
⇒
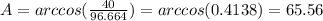
Therefore angle between u and v is 65.56°