Question

The fastest possible rate of rotation of a planet is that for which the gravitational force on material at the equator just barely provides the centripetal force needed for the rotation. (Why?)

(a) Show that the corresponding shortest period of rotation is T = √3π/Grho, where rho is the uniform density (mass per unit volume) of the spherical planet.
(b) Calculate the rotation period assuming a density of 3.0 g/cm³, typical of many planets, satellites, and asteroids. No astronomical object has ever been found to be spinning with a period shorter than that determined by this analysis.

Answer 1

6862.96871 seconds

Step-by-step explanation:

M = Mass of Planet

G = Gravitational constant

r = Radius

`\rho` = Density

T = Rotation period

In this system the gravitational force will balance the centripetal force

`G(Mm)/(r^2)=mr\omega^2`

`\omega=(2\pi)/(T)`.

`M=\rho v\\\Rightarrow M=\rho (4)/(3)\pi r^3`

`\\\Rightarrow G(Mm)/(r^2)=mr\left((2\pi)/(T)\right)^2\\\Rightarrow (G\rho (4)/(3)\pi r^3)/(r^3)=(4\pi^2)/(T^2)\\\Rightarrow T=\sqrt{(3\pi)/(G\rho)}`

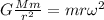
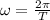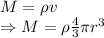
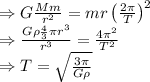
Hence, proved

`T=\sqrt{(3\pi)/(6.67* 10^(-11)* 3000)}\\\Rightarrow T=6862.96871\ s`

The rotation period of the astronomical object is 6862.96871 seconds