Question

Model rocket engines are rated by the impulse that they deliver when they fire. A particular engine is rated to deliver an impulse of 3.5 kg #m/s. The engine powers a 120 g rocket, including the mass of the engine. What is the final speed of the rocket once the engine has fired?

Answer 1

Final speed of the rocket,
`\Delta v= 29.16\ m/s`

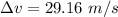
Step-by-step explanation:

It is given that,

Impulse delivered by an engine, J = 3.5 kg-m/s

Mass of the rocket, m = 120 g = 0.12 kg

To find,

The final speed of the rocket

Solution,

We know that the impulse is equal to the product of mass and velocity o it is equivalent to the change in momentum of an object.

`J=\Delta P=m* \Delta v`

`\Delta v=(J)/(m)`

`\Delta v=(3.5\ kg-m/s)/(0.12\ kg)`

`\Delta v= 29.16\ m/s`

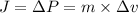
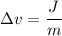
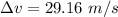
So, the final speed of the rocket once the engine has fired is 29.16 m/s.