Question

Find the point that is 2/3 the way from A to B where
A(1, 4) and B(4,-8).

Answer 1

The coordinates of the point M(x,y) = (3, -4)

Explanation:

The coordinates of the point A and B are A(1,4) and B(4,-8).

Let us assume the point which is 2/3 way on AB is m (x,y)

⇒ AM : AB = 2 : 3, So, AM = 2 parts of total 3 parts.

⇒ AM: MB = 2 : 1 (as M is 2 - 3rd )

Now, using Section Formula:

Coordinates for point (x,y) which vides the line (a,b) and (c,d) in m1: m2 is given as
`(x,y) = ((am2 + cm1)/(m1+m2) ,(dm1 + m2b)/(m1+m2) )`

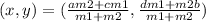
Applying this here on A(1,4) and B(4,-8) with m1: m2 = 2: 1

`(x,y) = ((1(1) + 2(4))/(2+1) ,(-8(2)+ 4(1))/(2+1) ) = ((1+8)/(3), (-16+4)/(3) )\\\implies (x,y) = ((9)/(3) ,(-12)/(3) )`

or, (x,y) = (3,-4)

Hence the coordinates of the point M(x,y) = (3, -4)