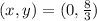Answer:
The coordinates of

Explanation:
The coordinates of the points are given as A(-2, 1) and B(4,6).
The ratio is 1 : 2
Le t us assume the point is M (x,y).
⇒ AM : MB = 1 : 2
Now, Using SECTION FORMULA:
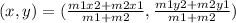
Using m1 : m2 = 1 : 2
Here, we get
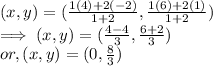
Hence, the coordinates of