Question

Mrs Wright spent 2/9 of her paycheck on food and 1/3 on rent. She spent 1/4 of the remainder on transportation. She had $210 left. How much was Mrs. Wright’s paycheck ?

Answer 1

Mrs Wright's paycheck was $ 630

Solution:

Given that,

Mrs Wright spend on food =
`(2)/(9)`

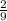
Fraction spent on rent =
`(1)/(3)`

She spent 1/4 of the remainder on transportation

Remaining left with her = $ 210

Let the paycheck amount be "n"

Now, we know that, paycheck amount = used amount + left over amount

Here, left over amount = 210

Paycheck amount = 2/9 of paycheck on food + 1/3 of paycheck on rent + 1/4 of remainder on transportation + 210

`n=(2)/(9) n+(1)/(3) n+(1)/(4)\left(n-(2)/(9) n-(1)/(3) n\right)+210`

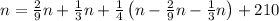
Upon simplifying we get,

`n=(2)/(9) n+(3)/(9) n+(1)/(4) n\left(1-(2)/(9)-(3)/(9)\right)+210`

`n=\left((2)/(9)+(3)/(9)\right) n+(1)/(4) n\left(1-((2+3))/(9)\right)+210`

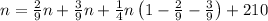
On simplification, we get

`n=(5)/(9) n+(1)/(4) n * (4)/(9)+210`

`\mathrm{n}=(5)/(9) \mathrm{n}+(1)/(9) \mathrm{n}+210`

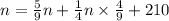
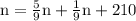
On adding fractions we get,

`\begin{array}{l}{\mathrm{n}=(6)/(9) \mathrm{n}+210} \\\\ {\mathrm{n}-(2)/(3) \mathrm{n}=210} \\\\ {(1)/(3) \mathrm{n}=210} \\\\ {\mathrm{n}=3 * 210=630}\end{array}`

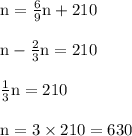
Hence, the paycheck amount is $630