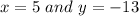The question is incomplete. Here is the complete question:
Find the values of x and y in the following equation. (x + yi) + (4 + 9i) = 9 -4i
A. x = 9 and y = -4
B. x = -9 and y = 4
C. x = 5 and y = -13
D. x = 5 and y = 13
Answer:
C.

Explanation:
Given:
The equation is given as:
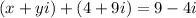
Let us combine the like terms using the commutative property of addition.
Combine the real parts together and imaginary parts together. This gives,

The left side of the equation is equal to the right side only if the real parts of both the sides are equal and imaginary parts of both the sides are equal. Therefore,
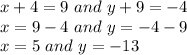
Therefore, the values of
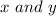 in the given equation is
in the given equation is