Question

5) James and Andrew are considering adding some premium channels to their satellite package. The cost of

their total bill if they added 1 and 4 premium channels is $45.60 and $66.60, respectively.

a) Find the slope.

b) Write the equation of the line.

c) Determine the base price (with O premium channels).

Answer 1

Part (A): The slope is 7.

Part (B): The required equation of line is
`y=7x+38.6`.

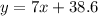Part (C): The base price is $38.6

Explanation:

Consider the provided information.

The cost of their total bill if they added 1 and 4 premium channels is $45.60 and $66.60, respectively.

For 1 premium channel they need to pay $45.60.

This can be written as: (1, 45.60)

For 4 premium channel they need to pay $66.60.

This can be written as: (4, 66.60)

Part (A) Find the slope.

`Slope=m=(y_2-y_1)/(x_2-x_1)`

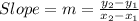
Substitute
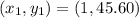`(x_1,y_1)=(1, 45.60)` and
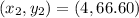`(x_2,y_2)=(4, 66.60)` in above formula.

`m=(66.60-45.60)/(4-1)`

`m=(21)/(3)=7`

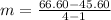
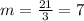
Hence, the slope is 7.

Part (B) Write the equation of the line.

By using one of the point and slope we can write the equation of line.

Point slope formula:
`(y-y_1)=m(x-x_1)`

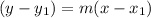
Substitute the respective values in the above formula.

`(y-45.60)=7(x-1)`

`y-45.60=7x-7`

`y=7x+38.6`

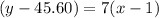
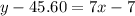
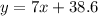
Hence, the required equation of line is
`y=7x+38.6`.

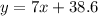Part (C) Determine the base price (with O premium channels)

Substitute x=0 in
`y=7x+38.6`.

`y=7(0)+38.6`

`y=38.6`

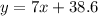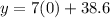
Hence, the base price is $38.6