Question

A thin Nichrome wire connected to an ammeter surrounds a region of time-varying magnetic flux, and the ammeter reads 13 amperes. If instead of a single wire we use a coil of thin Nichrome wire containing 23 turns, what does the ammeter read?

Answer 1

The current would be same in both situation.

Step-by-step explanation:

Given that,

Current I = 13 A

Number of turns = 23

We need to calculate the induced emf

Using formula of induced emf is

`\epsilon=NA(dB)/(dt)`

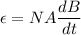
For N = 1

`\epsilon=A(dB)/(dt)`

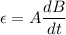
We need to calculate the current

Using formula of current

`i=(\epsilon)/(R)`

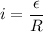
Put the value of emf

`i=(A(dB)/(dt))/(R)`

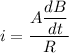
Now, if the number of turn is 22 , then induced emf would be

`\epsilon'=NA(dB)/(dt)`

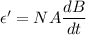
Then the current would be

`i'=(\epsilon')/(NR)`

`i'=(NA(dB)/(dt))/(NR)`

`i'=(A(dB)/(dt))/(R)`

`i'=i`

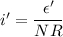
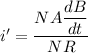
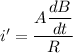
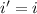
Hence, The current would be same in both situation.