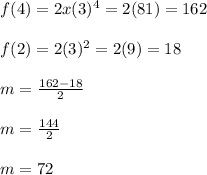Question

What is the average rate of change of the function f(x)=2(3)x from x = 2 to x = 4?

Enter your answer in the box.

Answer 1

The average rate of change of function is 72.

SOLUTION:

Given that, we have to find the average rate of change of the function f(x)=2(3)x from x = 2 to x = 4

Use the slope formula to find the average rate of change:

`\begin{array}{l}{m=(y 2-y 1)/(x 2-x 1)} \\\\ {m=(f(4)-f(2))/(4-2)} \\\\ {m=(f(4)-f(2))/(2)}\end{array}`

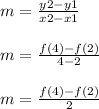
To simplify this, we need to find values of f ( 4 ) and f ( 2 ) :

`\begin{array}{l}{f(4)=2 x(3)^(4)=2(81)=162} \\\\ {f(2)=2(3)^(2)=2(9)=18} \\\\ {m=(162-18)/(2)} \\\\ {m=(144)/(2)} \\\\ {m=72}\end{array}`